Stochastics
The Stochastic (Greek for the art of guessing) is the mathematical discipline that deals with the random. Major motivations for its development were the calculation of odds for gambling, the question of fair operations and the question of a fair sharing of forces in broken gambling.
Nowadays results of Stochastics are applied in many very different fields: In the analysis and redesign of production processes (random influences here can bin in form of material errors), when interpreting the service at call centers (call times and call durations can be viewed as to be random), in the pattern and image recognition (distinct patterns are often disturbed by random noise), in the insurance industry (timing and extent of an accident or other insurance cases are considered to be randomly), in finance (equity and currency developments are considered as processes random), ...
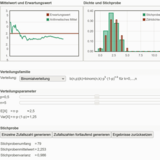
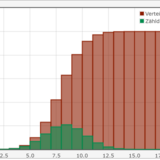
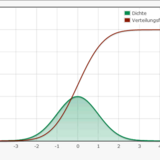
An essential object of the stochastics is to distinguish between different types of randomness: On a roll with a fair dice each of the 6 possible numbers remains with probability 1/6 above (equal distribution); if however, it is count how many times when you double-take with a fair coin "number" is above, it follows with probability 1/2 the value 1, while 0 and 2 respectively occur only with probability 1/4 (simplest form of a binomial distribution). A completely different type of randomness occurs when the life of a component is considered: Here, each non-negative real number is as the value in question. These different types of randomness lead to the concept of probability distributions.
For several such experiments, stochastic processes can be put together, which can be actually seen as modeling of real problems.
However, the concrete result of an experiment can not be predicted even with a known probability distribution. So one can never predict the individual result of a dice role or the lottery numbers from the next weekend. But long-term information is true, such that on repeated throwing a fair die, the proportion of fives in the long term tends to 1/6. Such statements are subject to limit theorems such as the strong law of large numbers or the central limit theorem.